
Write the S.I. unit of current density.
Answer
492.4k+ views
Hint:- Here in this question we should only focus on the current density or we can say current per unit area. And by keeping the formula of current density = \[\dfrac{{{\text{Total current}}}}{{{\text{Total Area}}}}\] in mind we can easily find out the S.I unit of current density.
Complete step-by-step answer:
As we know that the electric current is equal to the total charge flowing per unit time. So, the formula for electric current will be,
Current = \[\dfrac{{{\text{Total charge flowing}}}}{{{\text{Time}}}}\]
And current is denoted by I, charge is denoted by Q and time is denoted by T.
So, \[I = \dfrac{Q}{T}\] (1)
And the SI unit of the current is Ampere. Now let us understand the current density with the help of an example.
Let us say that the total current (I Amperes) is flowing within the conductor having cross-section area A.
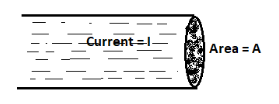
Now as we know that current density is equal to the total current per unit area.
So, current density = \[\dfrac{{{\text{Total current}}}}{{{\text{Total Area}}}}\]
As we know that current density is denoted by j.
So, now put all the values in the above equation. We get,
\[j = \dfrac{I}{{{\text{Area}}}}\] (2)
As we know that fundamental unit of current is Ampere and the fundamental unit of Area is \[{m^2}\]
So, now putting both the values of SI unit (i.e. SI unit of current and SI unit of area) in equation 2. We get,
\[j = \dfrac{A}{{{m^2}}}\]
On further solving we will get the SI unit of current density (j) as \[j = A{m^{ - 2}}\]
Hence, the SI unit of current density will be \[A{m^{ - 2}}\].
Note:- To solve this question we must keep in mind the basic formula of current i.e. (Current = \[\dfrac{{{\text{Total charge flowing}}}}{{{\text{Time}}}}\] ) and with this basic formula we will get the SI unit of current. So, if we directly put the SI unit of current and area in the formula of current density = \[\dfrac{{{\text{Total current}}}}{{{\text{Total Area}}}}\] we will get SI unit of current density.
Complete step-by-step answer:
As we know that the electric current is equal to the total charge flowing per unit time. So, the formula for electric current will be,
Current = \[\dfrac{{{\text{Total charge flowing}}}}{{{\text{Time}}}}\]
And current is denoted by I, charge is denoted by Q and time is denoted by T.
So, \[I = \dfrac{Q}{T}\] (1)
And the SI unit of the current is Ampere. Now let us understand the current density with the help of an example.
Let us say that the total current (I Amperes) is flowing within the conductor having cross-section area A.

Now as we know that current density is equal to the total current per unit area.
So, current density = \[\dfrac{{{\text{Total current}}}}{{{\text{Total Area}}}}\]
As we know that current density is denoted by j.
So, now put all the values in the above equation. We get,
\[j = \dfrac{I}{{{\text{Area}}}}\] (2)
As we know that fundamental unit of current is Ampere and the fundamental unit of Area is \[{m^2}\]
So, now putting both the values of SI unit (i.e. SI unit of current and SI unit of area) in equation 2. We get,
\[j = \dfrac{A}{{{m^2}}}\]
On further solving we will get the SI unit of current density (j) as \[j = A{m^{ - 2}}\]
Hence, the SI unit of current density will be \[A{m^{ - 2}}\].
Note:- To solve this question we must keep in mind the basic formula of current i.e. (Current = \[\dfrac{{{\text{Total charge flowing}}}}{{{\text{Time}}}}\] ) and with this basic formula we will get the SI unit of current. So, if we directly put the SI unit of current and area in the formula of current density = \[\dfrac{{{\text{Total current}}}}{{{\text{Total Area}}}}\] we will get SI unit of current density.
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

Which animal never drinks water in its entire life class 12 biology CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What is the potential difference Explain and give its class 12 physics CBSE




