
The length of a rectangle is 4 meters more than its breadth. What is its perimeter?.
Answer
506.1k+ views
Hint: We are asked to find the perimeter of a rectangle. First, we will find the dimension of the rectangle that means length and breadth. We will assume the breadth as x. So, as we have the length is 4 more than the breadth, so we will get length as 4 + x. Then we know that the perimeter is the sum of all the sides of the rectangle. So, we add both the length and breadth of the rectangle to get our radius.
Complete step-by-step answer:
We are asked to find the perimeter of a rectangle. We are given that the length is 4 more than its breadth. To find the perimeter, we have to first find the dimension of the rectangle. Let us assume that the breadth of the rectangle is x.
Breadth of rectangle = x meters…….(i)
Now, we get the breadth of the rectangle. We will look for the length of the rectangle. We are given that the length of the rectangle is 4 meters more than the breadth. As our breadth is x meters, so 4 meters more than the breadth means 4 more than x. So, the length of the rectangle is 4 more than x.
Length of rectangle = 4 + x meters
Now, we have the length as 4 + x and breadth as x. Now, we will find the perimeter of the rectangle. We know that the perimeter of the rectangle is given as the sum of all the sides.
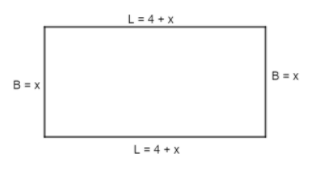
So, we get the perimeter of the rectangle as,
\[\text{Perimeter of rectangle}=x+4+x+x+4+x\]
\[\Rightarrow \text{Perimeter of rectangle}=4x+4+4\]
On simplifying further, we get,
\[\Rightarrow \text{Perimeter of rectangle}=4x+8\]
Note: As we get the length as 4 + x and breadth as x, then we know that the perimeter of the rectangle is given as 2 (L + B).
\[\text{Perimeter}=2\left( L+B \right)\]
\[\Rightarrow \text{Perimeter}=2\left( x+4+x \right)\]
On solving, we get,
\[\Rightarrow \text{Perimeter}=2\left( 2x+4 \right)\]
\[\Rightarrow \text{Perimeter}=4x+8\]
Remember that 4 more than something means 4 is added to it. 4 more than a thing means we have to multiply 4 with that.
Complete step-by-step answer:
We are asked to find the perimeter of a rectangle. We are given that the length is 4 more than its breadth. To find the perimeter, we have to first find the dimension of the rectangle. Let us assume that the breadth of the rectangle is x.
Breadth of rectangle = x meters…….(i)
Now, we get the breadth of the rectangle. We will look for the length of the rectangle. We are given that the length of the rectangle is 4 meters more than the breadth. As our breadth is x meters, so 4 meters more than the breadth means 4 more than x. So, the length of the rectangle is 4 more than x.
Length of rectangle = 4 + x meters
Now, we have the length as 4 + x and breadth as x. Now, we will find the perimeter of the rectangle. We know that the perimeter of the rectangle is given as the sum of all the sides.

So, we get the perimeter of the rectangle as,
\[\text{Perimeter of rectangle}=x+4+x+x+4+x\]
\[\Rightarrow \text{Perimeter of rectangle}=4x+4+4\]
On simplifying further, we get,
\[\Rightarrow \text{Perimeter of rectangle}=4x+8\]
Note: As we get the length as 4 + x and breadth as x, then we know that the perimeter of the rectangle is given as 2 (L + B).
\[\text{Perimeter}=2\left( L+B \right)\]
\[\Rightarrow \text{Perimeter}=2\left( x+4+x \right)\]
On solving, we get,
\[\Rightarrow \text{Perimeter}=2\left( 2x+4 \right)\]
\[\Rightarrow \text{Perimeter}=4x+8\]
Remember that 4 more than something means 4 is added to it. 4 more than a thing means we have to multiply 4 with that.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Why are resources distributed unequally over the e class 7 social science CBSE

Find the largest number which divides 615 and 963 leaving class 7 maths CBSE

One lakh eight thousand how can we write it in num class 7 maths CBSE

What is meant by Indian Standard Time Why do we need class 7 social science CBSE





