Question

The edge of an aluminium cube is 10cm long. One face of the cube is firmly fixed to a vertical wall. A mass of 100kg is then attached to the opposite face of the cube. The shear modulus of aluminium is 25GPa. What is the vertical deflection of this face?

Answer 1

Hint: By making a rough diagram you will understand that the tangential force caused due to the weight of the 10kg mass attached results in the mentioned vertical deflection. Recall the expression for shear modulus. You could rearrange the terms to get the vertical deflection caused. Then, you could directly substitute the given values to get the answer.

Formula used:
Expression for shear modulus,
$G=\dfrac{F\times L}{A\times \Delta x}$

Complete step by step answer:
In the question, we are given an aluminium cube of edge length 10cm with one of its faces fixed to a vertical wall and 100kg mass attached to the opposite face. We are also given the shear modulus of aluminium and are asked to find the vertical deflection of that face. The situation is clearly pictured below.

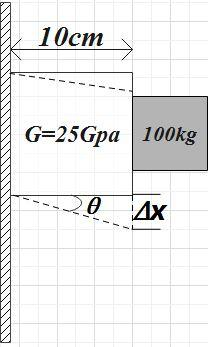
We know that the shear modulus is defined as the ratio of shearing stress to the corresponding shearing strain. It is normally represented by ‘G’. It is also known as the modulus of rigidity. So the shear modulus is given by,
$G=\dfrac{{{\sigma }_{s}}}{\tan \theta }$ ……………………. (1)
Where, ‘${{\sigma }_{s}}$’ is the shear stress and ‘$\tan \theta $’ gives the shear strain.
Shear stress just like any other stresses is the restoring force F acting per unit area but here the stress is caused as the result of applied tangential force, therefore,
${{\sigma }_{s}}=\dfrac{F}{A}$ ……………………… (2)
Shearing strain can be defined as the ratio of relative displacement of the faces to the original length, therefore,
$\tan \theta =\dfrac{\Delta x}{L}$ ……………………….. (3)
Substituting (2) and (3) in (1), we get,
$G=\dfrac{\dfrac{F}{A}}{\dfrac{\Delta x}{L}}$
$\Rightarrow \Delta x=\dfrac{F\times L}{A\times G}$ …………………………. (4)
Here $\Delta x$ the vertical deflection of the face.
The tangential force F is caused due the weight of the 100kg mass attached to the cube, therefore,
$F=mg=100\times 9.8=980N$
Area A is the area of the cube given by,
$A=\left( 10\times 10 \right)c{{m}^{2}}=100c{{m}^{2}}=0.01{{m}^{2}}$
Substituting all these values in (4) we get,
$\Delta x=\dfrac{980N\times 0.1m}{0.01{{m}^{2}}\times \left( 25\times {{10}^{9}} \right)pa}$
$\therefore \Delta x=392\times {{10}^{-9}}m$
Therefore, the vertical deflection of this face is $\Delta x=392nm$

Note:
While dealing with numerical problems, make sure that you convert all the given quantities into their SI units. Doing so makes calculation easier as well as could avoid further confusion. From the name of the modulus given in the question, you could easily get that the tangential force is causing stress and strain. So, always try to focus on such minute details in the question.