
Answer
427.9k+ views
Hint: Plot the point P (2, 3) on a Cartesian plane. Find the shortest distance this point places from the x – axis. Find the distance using the distance formula.
Complete step-by-step answer:
Let us mark the point P (2, 3) in the Cartesian plane.
The shortest distance between the coordinate (2, 3) and the x – axis is a straight line to the point (2, 0).
We are said to find the shortest distance from (x - axis). So the point is (2, 0).
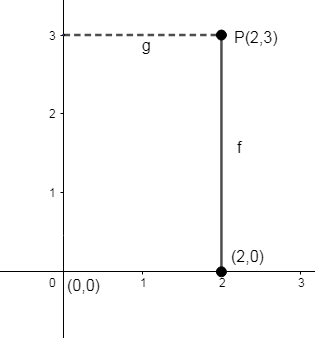
To find the distance, we can use the distance formula.
Distance formula \[=\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}\] Here, \[\left( {{x}_{1}},{{y}_{1}} \right)=\left( 2,0 \right)\], \[\left( {{x}_{2}},{{y}_{2}} \right)=\left( 2,3 \right)\].
\[\begin{align}
& =\sqrt{{{\left( 2-2 \right)}^{2}}+{{\left( 3-0 \right)}^{2}}} \\
& =\sqrt{0+9}=\sqrt{9} \\
& =3 \\
\end{align}\]
Thus the shortest distance of the point P (2, 3) to the x – axis is 3 units.
\[\therefore \] Option (b) is the correct answer.
Note: If we were asked to find the distance of point P (2, 3) from the y – axis, then the shortest distance between the coordinates (2, 3) and the y – axis will be a straight line to the point (0, 3).
Thus the distance can be calculated by distance formula.
\[\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}=\sqrt{{{\left( 2-0 \right)}^{2}}+{{\left( 3-3 \right)}^{2}}}=\sqrt{4+0}=\sqrt{4}=2\] units.
\[\therefore \] The shortest distance of the point P (2, 3) to the y – axis is 2 units.
Complete step-by-step answer:
Let us mark the point P (2, 3) in the Cartesian plane.
The shortest distance between the coordinate (2, 3) and the x – axis is a straight line to the point (2, 0).
We are said to find the shortest distance from (x - axis). So the point is (2, 0).

To find the distance, we can use the distance formula.
Distance formula \[=\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}\] Here, \[\left( {{x}_{1}},{{y}_{1}} \right)=\left( 2,0 \right)\], \[\left( {{x}_{2}},{{y}_{2}} \right)=\left( 2,3 \right)\].
\[\begin{align}
& =\sqrt{{{\left( 2-2 \right)}^{2}}+{{\left( 3-0 \right)}^{2}}} \\
& =\sqrt{0+9}=\sqrt{9} \\
& =3 \\
\end{align}\]
Thus the shortest distance of the point P (2, 3) to the x – axis is 3 units.
\[\therefore \] Option (b) is the correct answer.
Note: If we were asked to find the distance of point P (2, 3) from the y – axis, then the shortest distance between the coordinates (2, 3) and the y – axis will be a straight line to the point (0, 3).
Thus the distance can be calculated by distance formula.
\[\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}=\sqrt{{{\left( 2-0 \right)}^{2}}+{{\left( 3-3 \right)}^{2}}}=\sqrt{4+0}=\sqrt{4}=2\] units.
\[\therefore \] The shortest distance of the point P (2, 3) to the y – axis is 2 units.
Recently Updated Pages
Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 Maths: Engaging Questions & Answers for Success

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Trending doubts
Name the states which share their boundary with Indias class 9 social science CBSE

Explain the necessity of Political Parties in a de class 9 social science CBSE

What was the main aim of the Treaty of Vienna of 1 class 9 social science CBSE

Differentiate between nationstate and modern state class 9 social science CBSE

Find the equation of the circle passing through the class 9 maths CBSE

Evaluate the powersharing system in India class 9 social science CBSE




