
PQ is a tangent to a circle with centre O at the point P. If \[\vartriangle OPQ\] is an isosceles triangle with P as a vertex , then \[\angle OQP\] is equal to
a) \[{30^ \circ }\]
b) \[{45^ \circ }\]
c) \[{60^ \circ }\]
d) \[{90^ \circ }\]
Answer
457.2k+ views
Hint: We are given that PQ is a tangent to a circle with centre O at the point P. Let us consider OP to be the radius of the circle. Then, as PQ is tangent at point P, we know the tangent makes a right angle with the radius. Hence, \[\angle OPQ = {90^ \circ }\]. Also, we are given that \[\vartriangle OPQ\] is an isosceles triangle. We will understand this using a diagram.
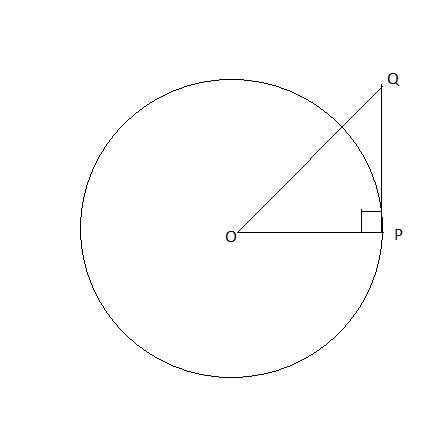
In the given figure, OP is the radius of the circle and PQ is tangent at point P. Hence, \[\angle OPQ = {90^ \circ }\]. Since \[\vartriangle OPQ\] is an isosceles triangle, we have two sides of this triangle are equal. Let us consider \[OP = PQ\]. Now, we will use the property of an isosceles triangle that angles opposite to equal sides are equal. After that we will use the angle sum property of a triangle i.e. Sum of angles in a triangle is equal to \[{180^ \circ }\] and find the required angle.
Complete step-by-step solution:
Since PQ is a tangent at point P and OP is the radius of the circle.
We see it through a figure.
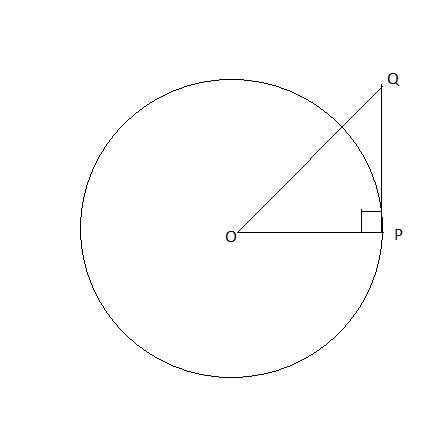
We know, tangent makes a right angle with the radius and So,
\[\angle OPQ = {90^ \circ } - - - - - (1)\]
As \[\vartriangle OPQ\] is an isosceles triangle with \[OP = PQ\].
We know, Angles opposite to equal sides are equal.
Angle opposite to \[PQ\] is \[\angle QOP\].
Angle opposite to \[OP\] is \[\angle OQP\].
Hence, \[\angle OQP = \angle QOP - - - - - (2)\]
In \[\vartriangle OPQ\], using Angle Sum Property
\[\angle OPQ + \angle OQP + \angle QOP = {180^ \circ } - - - - - (3)\]
Using (1) and (2) in (3)
\[{90^ \circ } + \angle OQP + \angle OQP = {180^ \circ }\]
\[ \Rightarrow {90^ \circ } + 2\angle OQP = {180^ \circ }\]
Subtracting \[{90^ \circ }\] both the sides, we get
\[ \Rightarrow {90^ \circ } + 2\angle OQP - {90^ \circ } = {180^ \circ } - {90^ \circ }\]
Clubbing the like terms on left hand side
\[ \Rightarrow 2\angle OQP + ({90^ \circ } - {90^ \circ }) = {180^ \circ } - {90^ \circ }\]
Solving the left and the right hand side
\[ \Rightarrow 2\angle OQP + {0^ \circ } = {90^ \circ }\]
\[ \Rightarrow 2\angle OQP = {90^ \circ }\]
Dividing both the sides by \[2\].
\[\dfrac{{2\angle OQP}}{2} = \dfrac{{{{90}^ \circ }}}{2}\]
\[\angle OQP = {45^ \circ }\]
Hence, we got \[\angle OQP = {45^ \circ }\].
Therefore, the correct option is (b).
Note: We need to be very thorough with the properties of circles and triangles. Once we interpret the given question, we need to think of the steps of solving the problem. While solving, we need to take care of the calculations. We have to read the question carefully and then draw the figure and then apply the properties. Each and every detail in the question needs to be read very carefully. While solving, we have to take care of each and every step.

In the given figure, OP is the radius of the circle and PQ is tangent at point P. Hence, \[\angle OPQ = {90^ \circ }\]. Since \[\vartriangle OPQ\] is an isosceles triangle, we have two sides of this triangle are equal. Let us consider \[OP = PQ\]. Now, we will use the property of an isosceles triangle that angles opposite to equal sides are equal. After that we will use the angle sum property of a triangle i.e. Sum of angles in a triangle is equal to \[{180^ \circ }\] and find the required angle.
Complete step-by-step solution:
Since PQ is a tangent at point P and OP is the radius of the circle.
We see it through a figure.

We know, tangent makes a right angle with the radius and So,
\[\angle OPQ = {90^ \circ } - - - - - (1)\]
As \[\vartriangle OPQ\] is an isosceles triangle with \[OP = PQ\].
We know, Angles opposite to equal sides are equal.
Angle opposite to \[PQ\] is \[\angle QOP\].
Angle opposite to \[OP\] is \[\angle OQP\].
Hence, \[\angle OQP = \angle QOP - - - - - (2)\]
In \[\vartriangle OPQ\], using Angle Sum Property
\[\angle OPQ + \angle OQP + \angle QOP = {180^ \circ } - - - - - (3)\]
Using (1) and (2) in (3)
\[{90^ \circ } + \angle OQP + \angle OQP = {180^ \circ }\]
\[ \Rightarrow {90^ \circ } + 2\angle OQP = {180^ \circ }\]
Subtracting \[{90^ \circ }\] both the sides, we get
\[ \Rightarrow {90^ \circ } + 2\angle OQP - {90^ \circ } = {180^ \circ } - {90^ \circ }\]
Clubbing the like terms on left hand side
\[ \Rightarrow 2\angle OQP + ({90^ \circ } - {90^ \circ }) = {180^ \circ } - {90^ \circ }\]
Solving the left and the right hand side
\[ \Rightarrow 2\angle OQP + {0^ \circ } = {90^ \circ }\]
\[ \Rightarrow 2\angle OQP = {90^ \circ }\]
Dividing both the sides by \[2\].
\[\dfrac{{2\angle OQP}}{2} = \dfrac{{{{90}^ \circ }}}{2}\]
\[\angle OQP = {45^ \circ }\]
Hence, we got \[\angle OQP = {45^ \circ }\].
Therefore, the correct option is (b).
Note: We need to be very thorough with the properties of circles and triangles. Once we interpret the given question, we need to think of the steps of solving the problem. While solving, we need to take care of the calculations. We have to read the question carefully and then draw the figure and then apply the properties. Each and every detail in the question needs to be read very carefully. While solving, we have to take care of each and every step.
Recently Updated Pages
Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 10 Science: Engaging Questions & Answers for Success

Trending doubts
Who was appointed as the first chairman of the Indian class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Acids are always stored in containers made of A plastic class 10 chemistry CBSE

Five things I will do to build a great India class 10 english CBSE

Ladang is the name given to shifting cultivation in class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths




