
If v is the image distance and u is the object distance, then the lateral magnification (m) is given as:
A. \[m=\dfrac{v}{u}\]
B. \[m=\dfrac{v+u}{v-u}\]
C. \[m=\dfrac{u}{v}\]
D. \[m=\dfrac{v-u}{v+u}\]
Answer
515.1k+ views
Hint: In this question we have been asked to derive the formula for lateral magnification. The image distance and object distance are given as v and u respectively. Therefore, to solve this question, we shall draw a ray diagram to derive the formula for lateral magnification. Lateral magnification is given as the ratio of image height over the object height.
Complete step by step solution:
Consider the following ray diagram.
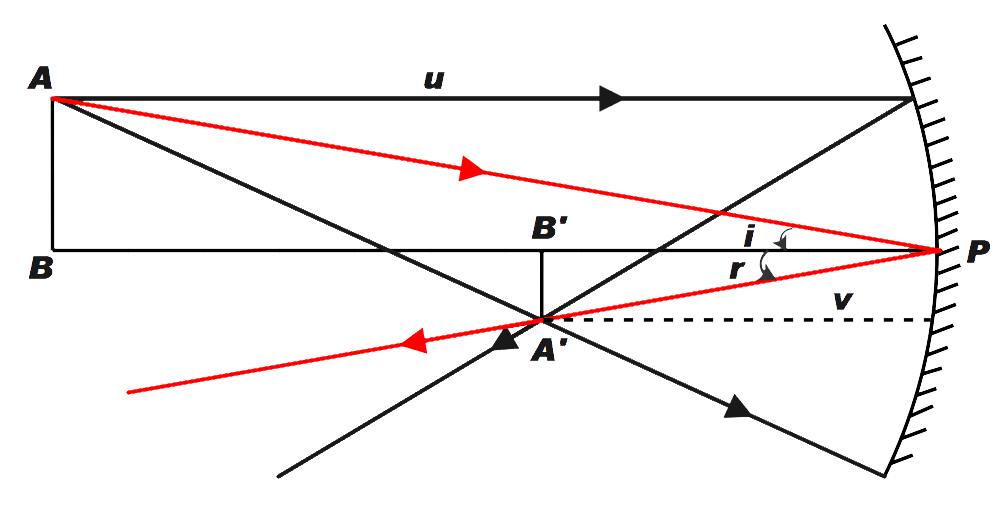
In the diagram above, v is the image distance and u are the object distance from the mirror. From the diagram we can see that the image formed is inverted.
Let,
A’B’ be the image height \[{{h}_{i}}\] and AB be the object height \[{{h}_{o}}\]
We know that lateral magnification is given as the ratio of image height over the object height.
Therefore,
\[m=\dfrac{A'B'}{AB}=\dfrac{{{h}_{i}}}{{{h}_{o}}}\] …………. (1)
Now, consider \[\vartriangle ABP\] and \[\vartriangle A'B'P\]
In \[\vartriangle ABP\],
\[\angle APB\] is the angle of incidence and \[\angle A'PB'\] is the angle of reflection.
We know that angle of incidence is equal to the angle of reflection
Therefore, we can say that,
\[\vartriangle ABP\sim \vartriangle A'B'P\]
Therefore, from similar triangle properties we can say that,
\[\dfrac{A'B'}{AB}=\dfrac{B'P}{BP}\]
Now, from the diagram above
We get,
\[\dfrac{B'P}{BP}=\dfrac{v}{u}\] ………………….. (2)
Now, from (1) and (2)
We get,
\[m=\dfrac{{{h}_{i}}}{{{h}_{o}}}=\dfrac{v}{u}\]
Therefore,
\[m=\dfrac{v}{u}\]
So, the correct answer is “Option A”.
Note: Magnification is the process of obtaining the enlarged image of the given object. Lateral magnification refers to enlarging the lateral image. The formula for lateral magnification gives the relation between the size of image and object as well as the distance of object and image from the mirror. When the magnification is negative the image obtained is inverted. Also, if the magnification is less than 1 the image size is diminished and vice versa.
Complete step by step solution:
Consider the following ray diagram.

In the diagram above, v is the image distance and u are the object distance from the mirror. From the diagram we can see that the image formed is inverted.
Let,
A’B’ be the image height \[{{h}_{i}}\] and AB be the object height \[{{h}_{o}}\]
We know that lateral magnification is given as the ratio of image height over the object height.
Therefore,
\[m=\dfrac{A'B'}{AB}=\dfrac{{{h}_{i}}}{{{h}_{o}}}\] …………. (1)
Now, consider \[\vartriangle ABP\] and \[\vartriangle A'B'P\]
In \[\vartriangle ABP\],
\[\angle APB\] is the angle of incidence and \[\angle A'PB'\] is the angle of reflection.
We know that angle of incidence is equal to the angle of reflection
Therefore, we can say that,
\[\vartriangle ABP\sim \vartriangle A'B'P\]
Therefore, from similar triangle properties we can say that,
\[\dfrac{A'B'}{AB}=\dfrac{B'P}{BP}\]
Now, from the diagram above
We get,
\[\dfrac{B'P}{BP}=\dfrac{v}{u}\] ………………….. (2)
Now, from (1) and (2)
We get,
\[m=\dfrac{{{h}_{i}}}{{{h}_{o}}}=\dfrac{v}{u}\]
Therefore,
\[m=\dfrac{v}{u}\]
So, the correct answer is “Option A”.
Note: Magnification is the process of obtaining the enlarged image of the given object. Lateral magnification refers to enlarging the lateral image. The formula for lateral magnification gives the relation between the size of image and object as well as the distance of object and image from the mirror. When the magnification is negative the image obtained is inverted. Also, if the magnification is less than 1 the image size is diminished and vice versa.
Recently Updated Pages
Full Form: Updated List (2025) of Important Abbreviations You Should Know

OTG Full Form: Oven Toaster Grill & On-The-Go Explained

IT Full Form: Information Technology Explained for Students

ISO Full Form: Meaning, Certification, Uses & Examples

OPD Full Form in Medical: Meaning, Difference & Uses (2025)

ISD Full Form: Meaning in Telecom, Education, GST & More

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Who discovered the cell and how class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE




