
How do you find related acute angles?
Answer
448.8k+ views
Hint: since we have been told to find the related acute angle in the question, try to define acute angle and related acute angle first. You will get to know the difference between both of them and hence it will further make it easy to find the related acute angle from any measurement of angle.
Complete step by step answer:
An angle which is $ < {90^\circ }$ (less than 90) is called a cute angle. Now a related acute angle is defined as the acute angle which is formed by the x-axis and the terminal side only when the terminal side is in the 2-quadrant, 3-quadrant and the 4-quadrant. A terminal side is the other side of the angle the other side of the angle other than the fixed side of an angle.
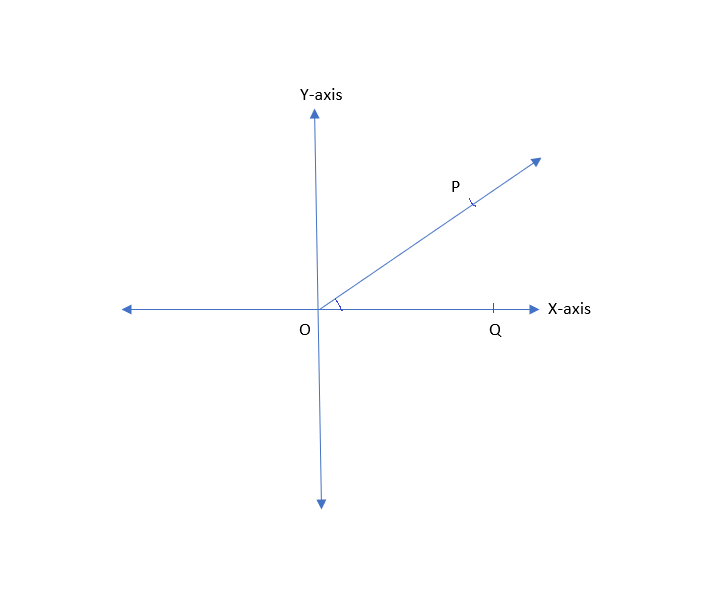
For example side PO is the terminal side of $\angle POQ$ and side OQ is the fixed side in the above figure.
Now the principal angle is the angle formed by the positive x-axis and the terminal side in an anticlockwise direction. To find the related acute angle one should follow the given steps:
1.If the terminal side lies in the 2-quadrant, subtract the principle angle from ${180^\circ }$. This will give us the related acute angle.
2.If the terminal side lies in the 3-quadrant, subtract ${180^\circ }$ from the principal angle.
3.If the terminal side lies in the 4-quadrant, subtract the principle angle from ${360^\circ }$ to find the related acute angle.
Note: For solving these types of questions the only thing you need to know is the definition correctly. The value of the related acute angle will always measure between ${0^\circ }$ and ${90^\circ }$ even if it is present in any of the four quadrants. If the related acute angle is counted in an anti-clockwise direction it will always be positive.
Complete step by step answer:
An angle which is $ < {90^\circ }$ (less than 90) is called a cute angle. Now a related acute angle is defined as the acute angle which is formed by the x-axis and the terminal side only when the terminal side is in the 2-quadrant, 3-quadrant and the 4-quadrant. A terminal side is the other side of the angle the other side of the angle other than the fixed side of an angle.

For example side PO is the terminal side of $\angle POQ$ and side OQ is the fixed side in the above figure.
Now the principal angle is the angle formed by the positive x-axis and the terminal side in an anticlockwise direction. To find the related acute angle one should follow the given steps:
1.If the terminal side lies in the 2-quadrant, subtract the principle angle from ${180^\circ }$. This will give us the related acute angle.
2.If the terminal side lies in the 3-quadrant, subtract ${180^\circ }$ from the principal angle.
3.If the terminal side lies in the 4-quadrant, subtract the principle angle from ${360^\circ }$ to find the related acute angle.
Note: For solving these types of questions the only thing you need to know is the definition correctly. The value of the related acute angle will always measure between ${0^\circ }$ and ${90^\circ }$ even if it is present in any of the four quadrants. If the related acute angle is counted in an anti-clockwise direction it will always be positive.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

What were the major teachings of Baba Guru Nanak class 7 social science CBSE





