
Answer
429.6k+ views
Hint:
Here, we will compare the given equation with the general equation of parabola to find the vertex. Then we will find \[x\] and \[y\] intercepts and also an additional point by substituting different values of the variables in the vertex form of the given equation. Using this we will plot those points on a graph and join them together to find the required graph of the given parabola.
Complete step by step solution:
The given quadratic equation in vertex form is $y = - 3{\left( {x - 2} \right)^2} + 5$
Comparing this with $y = a{\left( {x - h} \right)^2} + k$, where $a$ is the axis of symmetry and $\left( {h,k} \right)$ is the vertex.
Now, in order to graph a parabola of this quadratic equation, first of all, we will find the vertex.
Now, since, according to the question, $a = - 3 < 0$ therefore, the vertex will be at the maximum point and the parabola will open downwards.
Thus, comparing the given equation with the general equation, we have,
Vertex $\left( {h,k} \right) = \left( {2,5} \right)$
Now, substituting $x = 0$ in the given quadratic equation $y = - 3{\left( {x - 2} \right)^2} + 5$, we get,
$ y = - 3{\left( {0 - 2} \right)^2} + 5$
$ \Rightarrow y = - 3 \times 4 + 5 = - 12 + 5 = - 7$
Hence, when $x = 0$, $y = - 7$.
Therefore, the $y$-intercept is $\left( {0, - 7} \right)$
Similarly, substituting $y = 0$ in the given quadratic equation $y = - 3{\left( {x - 2} \right)^2} + 5$, we get,
$ 0 = - 3{\left( {x - 2} \right)^2} + 5$
$ \Rightarrow 3{\left( {x - 2} \right)^2} = 5$
Dividing both sides by 3, we get
$ \Rightarrow {\left( {x - 2} \right)^2} = \dfrac{5}{3}$
Taking square root on both sides, we gt
$ \Rightarrow x - 2 = \pm \sqrt {\dfrac{5}{3}} $
Adding 2 on both sides, we get,
$ \Rightarrow x = 2 \pm \dfrac{{\sqrt 5 }}{{\sqrt 3 }}$
Hence, when $y = 0$, $x = 2 + \dfrac{{\sqrt 5 }}{{\sqrt 3 }},2 - \dfrac{{\sqrt 5 }}{{\sqrt 3 }}$
Therefore, the $x$-intercepts are $\left( {2 + \dfrac{{\sqrt 5 }}{{\sqrt 3 }},0} \right)$ and $\left( {2 - \dfrac{{\sqrt 5 }}{{\sqrt 3 }},0} \right)$
If we do the approximate then, the $x$-intercepts are $\left( { \approx 3.291,0} \right)$ and $\left( {0.709,0} \right)$
Now, we will find an additional point also.
Thus, let $x = 4$
Hence, we get,
$y = - 3{\left( {4 - 2} \right)^2} + 5$
$ \Rightarrow y = - 3 \times 4 + 5 = - 12 + 5 = - 7$
Hence, additional point is $\left( {4, - 7} \right)$
Now, we will draw the graph of parabola, such that,
Its vertex is $\left( {2,5} \right)$
The $x$-intercepts are $\left( { \approx 3.291,0} \right)$ and $\left( {0.709,0} \right)$
The $y$-intercept is $\left( {0, - 7} \right)$
An additional point is $\left( {4, - 7} \right)$
Hence, the required graph is:
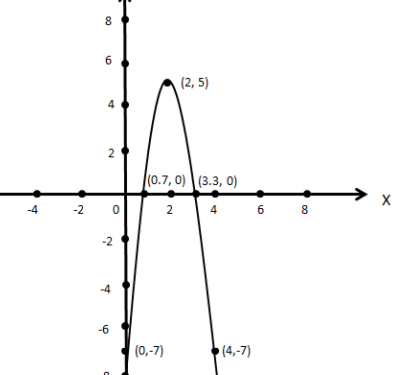
Hence, this is the required answer.
Note:
A parabola is a curve having a focus and a directrix, such that each point on parabola is at equal distance from them. The axis of symmetry of a parabola is a line about which the parabola is symmetrical. When the parabola is vertical, the line of symmetry is vertical. When a quadratic function is graphed in the coordinate plane, the resulting parabola and corresponding axis of symmetry are vertical.
Here, we will compare the given equation with the general equation of parabola to find the vertex. Then we will find \[x\] and \[y\] intercepts and also an additional point by substituting different values of the variables in the vertex form of the given equation. Using this we will plot those points on a graph and join them together to find the required graph of the given parabola.
Complete step by step solution:
The given quadratic equation in vertex form is $y = - 3{\left( {x - 2} \right)^2} + 5$
Comparing this with $y = a{\left( {x - h} \right)^2} + k$, where $a$ is the axis of symmetry and $\left( {h,k} \right)$ is the vertex.
Now, in order to graph a parabola of this quadratic equation, first of all, we will find the vertex.
Now, since, according to the question, $a = - 3 < 0$ therefore, the vertex will be at the maximum point and the parabola will open downwards.
Thus, comparing the given equation with the general equation, we have,
Vertex $\left( {h,k} \right) = \left( {2,5} \right)$
Now, substituting $x = 0$ in the given quadratic equation $y = - 3{\left( {x - 2} \right)^2} + 5$, we get,
$ y = - 3{\left( {0 - 2} \right)^2} + 5$
$ \Rightarrow y = - 3 \times 4 + 5 = - 12 + 5 = - 7$
Hence, when $x = 0$, $y = - 7$.
Therefore, the $y$-intercept is $\left( {0, - 7} \right)$
Similarly, substituting $y = 0$ in the given quadratic equation $y = - 3{\left( {x - 2} \right)^2} + 5$, we get,
$ 0 = - 3{\left( {x - 2} \right)^2} + 5$
$ \Rightarrow 3{\left( {x - 2} \right)^2} = 5$
Dividing both sides by 3, we get
$ \Rightarrow {\left( {x - 2} \right)^2} = \dfrac{5}{3}$
Taking square root on both sides, we gt
$ \Rightarrow x - 2 = \pm \sqrt {\dfrac{5}{3}} $
Adding 2 on both sides, we get,
$ \Rightarrow x = 2 \pm \dfrac{{\sqrt 5 }}{{\sqrt 3 }}$
Hence, when $y = 0$, $x = 2 + \dfrac{{\sqrt 5 }}{{\sqrt 3 }},2 - \dfrac{{\sqrt 5 }}{{\sqrt 3 }}$
Therefore, the $x$-intercepts are $\left( {2 + \dfrac{{\sqrt 5 }}{{\sqrt 3 }},0} \right)$ and $\left( {2 - \dfrac{{\sqrt 5 }}{{\sqrt 3 }},0} \right)$
If we do the approximate then, the $x$-intercepts are $\left( { \approx 3.291,0} \right)$ and $\left( {0.709,0} \right)$
Now, we will find an additional point also.
Thus, let $x = 4$
Hence, we get,
$y = - 3{\left( {4 - 2} \right)^2} + 5$
$ \Rightarrow y = - 3 \times 4 + 5 = - 12 + 5 = - 7$
Hence, additional point is $\left( {4, - 7} \right)$
Now, we will draw the graph of parabola, such that,
Its vertex is $\left( {2,5} \right)$
The $x$-intercepts are $\left( { \approx 3.291,0} \right)$ and $\left( {0.709,0} \right)$
The $y$-intercept is $\left( {0, - 7} \right)$
An additional point is $\left( {4, - 7} \right)$
Hence, the required graph is:

Hence, this is the required answer.
Note:
A parabola is a curve having a focus and a directrix, such that each point on parabola is at equal distance from them. The axis of symmetry of a parabola is a line about which the parabola is symmetrical. When the parabola is vertical, the line of symmetry is vertical. When a quadratic function is graphed in the coordinate plane, the resulting parabola and corresponding axis of symmetry are vertical.
Recently Updated Pages
A particle is undergoing a horizontal circle of radius class 11 physics CBSE

A particle is thrown vertically upwards with a velocity class 11 physics CBSE

A particle is rotated in a vertical circle by connecting class 11 physics CBSE

A particle is projected with a velocity v such that class 11 physics CBSE

A particle is projected with a velocity u making an class 11 physics CBSE

A particle is projected vertically upwards and it reaches class 11 physics CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE




