Question

A starts from a place P to go to a place Q. at the same time B starts from Q to P. if after meeting each other, A and B took 4 hours and 9 hours more respectively to reach their destination, what is the ratio of their speeds?
(a) 3:2
(b) 5:2
(c) 9:4
(d) 9:13

Answer 1

Hint: To solve this question, we will consider a point C’ between places P and Q where A and B meet. We will consider the distance PC as x and CQ as y. After this, we will apply the condition that at the same time A and B course different distances. Also, we will apply the condition of the remaining distances for A and Band how much time they need to cover it.

Complete step-by-step answer:
To solve this question, we will consider here a point C between the points P and Q such that the total distance between P and Q is d as shown:

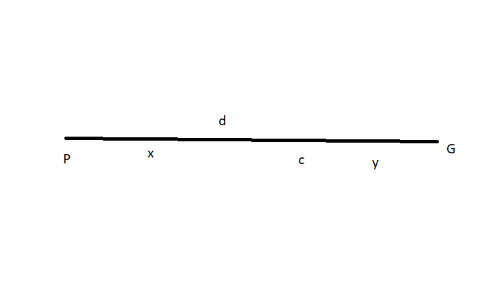
Thus, from above figure we can see that the total distance, d=x+y. therefore:
$\Rightarrow y=d-x...........(i)$
We are given that, now A and B have met at point C. it is further given that the time taken for A to go from point c to point Q is 4 hours. Let us say that the speed of A is ${{V}_{A}}$ then the relation between the distance travelled, velocity and time elapsed is given as
\[\begin{align}
  & speed=\dfrac{Total\,distance}{total\,time} \\
 & {{V}_{A}}=y \\
 & \Rightarrow y=4{{V}_{A}}............(ii) \\
\end{align}\]
Now, we are given that the time taken for B to go to point Q from point A is 9 hours. Thus, the relation between speed, distance travelled and total time taken is given by
$\begin{align}
  & {{V}_{B}}=\dfrac{x}{9} \\
 & \Rightarrow x=9{{V}_{B}}............(iii) \\
\end{align}$
Where ${{V}_{B}}$= velocity of B
Now, we are given that, initially A takes time t and reach the point C from point P. the same time is also taken by B to reach the point C from point Q. thus, we get the following relation:
$\begin{align}
  & x={{V}_{A}}t.............(iv) \\
 & y={{V}_{B}}t..............(v) \\
\end{align}$
Now we will divide the equation (iv) by equation (v). After dividing, we will get:
$\begin{align}
  & \dfrac{x}{y}=\dfrac{{{V}_{A}}t}{{{V}_{B}}t} \\
 & \Rightarrow \dfrac{x}{y}=\dfrac{{{V}_{A}}}{{{V}_{B}}}...........(vi) \\
\end{align}$
Now, we will put the values of x and y from equation (iv) and (v) into the equation (vi). After doing this, we will get following:
\[\dfrac{9{{V}_{B}}}{4{{V}_{A}}}=\dfrac{{{V}_{A}}}{{{V}_{B}}}\]
$\begin{align}
  & \Rightarrow 9{{V}_{B}}^{2}=4{{V}_{A}}^{2} \\
 & \Rightarrow 3{{V}_{B}}=2{{V}_{A}} \\
 & \Rightarrow \dfrac{{{V}_{A}}}{{{V}_{B}}}=\dfrac{3}{2} \\
\end{align}$

So, the correct answer is “Option A”.

Note: Another way of doing this question is as follows when A and B meet at point C, then we can have following relation:
\[{{V}_{A}}t+{{V}_{B}}t=d\]
After meeting, the distance left for $A={{V}_{B}}t$ and the distance left for $B={{V}_{A}}t$. Now according to the question, ${{V}_{B}}t={{V}_{A}}$. Also ${{V}_{A}}t=9{{V}_{B}}$. Therefore we get:
$\dfrac{{{V}_{B}}t}{{{V}_{A}}t}=\dfrac{4{{V}_{A}}}{9{{V}_{B}}}\Rightarrow \dfrac{{{V}_{A}}}{{{V}_{B}}}=\dfrac{3}{2}$