
A rectangular garden is $120m$ long and $85m$ broad. Find its area and perimeter.
Answer
480k+ views
Hint: We are given the length and breadth of the rectangular garden. We know the area of a rectangle is the product of its length and breadth. Also the perimeter of a rectangle is twice the sum of the length and the breadth. So substituting these we get the answers.
Useful formula:
For a rectangle of length $l$ and breadth $b$,
Area of the rectangle, $A = l \times b$
Perimeter of the rectangle, $P = 2(l + b)$
Complete step-by-step answer:
It is given that a rectangular garden is $120m$ long and $85m$ broad.
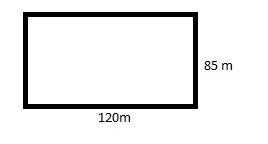
We are asked to find its area and perimeter.
For a rectangle of length $l$and breadth $b$,
Area of the rectangle, $A = l \times b$
Perimeter of the rectangle, $P = 2(l + b)$
Here, $l = 120m$, $b = 85m$
Substituting we get,
Area of the rectangle, $A = 120 \times 85 = 10200{m^2}$
Perimeter of the rectangle, $P = 2 \times (120 + 85) = 2 \times 205 = 410m$
So the area and perimeter of the rectangular garden are $10200{m^2}$ and $410m$ respectively.
Additional information:
A rectangle becomes a square if the length and breadth are the same.
Area of the square is the square of its side.
Perimeter of a square is four times the length of the side.
When one of the diagonals of a rectangle is drawn, we get two right angled triangles with half the area of the rectangle.
Note: Here we are using the formula of area and perimeter and directly substituting the length and the breadth. If the area is given and any of the length or breadth is known we can substitute it to find perimeter. So questions can be asked in different ways from the section area and perimeter.
Useful formula:
For a rectangle of length $l$ and breadth $b$,
Area of the rectangle, $A = l \times b$
Perimeter of the rectangle, $P = 2(l + b)$
Complete step-by-step answer:
It is given that a rectangular garden is $120m$ long and $85m$ broad.

We are asked to find its area and perimeter.
For a rectangle of length $l$and breadth $b$,
Area of the rectangle, $A = l \times b$
Perimeter of the rectangle, $P = 2(l + b)$
Here, $l = 120m$, $b = 85m$
Substituting we get,
Area of the rectangle, $A = 120 \times 85 = 10200{m^2}$
Perimeter of the rectangle, $P = 2 \times (120 + 85) = 2 \times 205 = 410m$
So the area and perimeter of the rectangular garden are $10200{m^2}$ and $410m$ respectively.
Additional information:
A rectangle becomes a square if the length and breadth are the same.
Area of the square is the square of its side.
Perimeter of a square is four times the length of the side.
When one of the diagonals of a rectangle is drawn, we get two right angled triangles with half the area of the rectangle.
Note: Here we are using the formula of area and perimeter and directly substituting the length and the breadth. If the area is given and any of the length or breadth is known we can substitute it to find perimeter. So questions can be asked in different ways from the section area and perimeter.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

What were the major teachings of Baba Guru Nanak class 7 social science CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





