
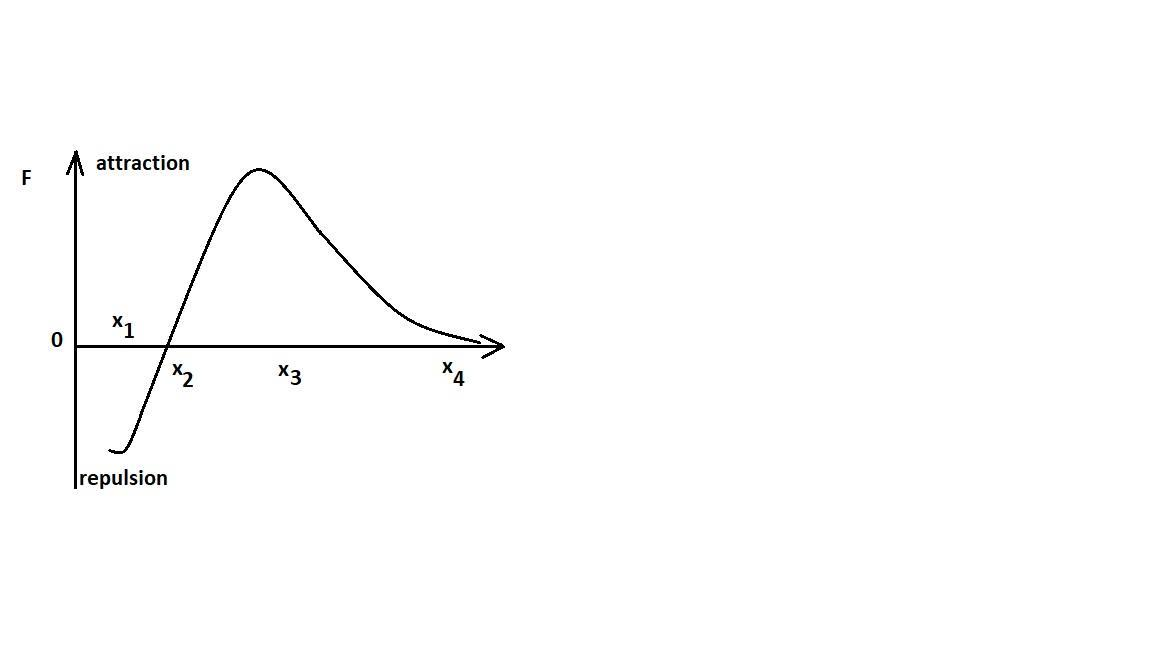
The diagram given shows how the net interaction force (conservative) between two particles $A$ and $B$ is related to the distance between them varies from \[{x_1}\] to \[{x_2}\] . Then
(A) Potential energy of the system increases from \[{x_1}\] to \[{x_2}\]
(B) Potential energy of the system increases from \[{x_2}\] to \[{x_3}\]
(C) Potential energy of the system increases from \[{x_3}\] to \[{x_4}\]
(D) KE increases from \[{x_1}\] to \[{x_2}\] and decreases from \[{x_2}\] to \[{x_3}\]
Answer
139.8k+ views
Hint: We will pull in the concept of potential energy to be the work done stored by the system. We will then try out the relationships and then finally find the correct option.
Formulae Used $F = - \frac{{dU}}{{dx}}$
Step By Step Solution
Firstly,
$F = - \frac{{dU}}{{dx}}$
Thus, we can say,
\[dU = - Fdx\]
Now,
Clearly,
When $F$ is positive, $dU$ is negative and when $F$ is negative, $dU$ is positive.
For the region from \[{x_1}\] to \[{x_2}\] , $F$ is negative as we can see from the graph itself.
Thus, $dU$ is positive.
Which means potential energy increases from \[{x_1}\] to \[{x_2}\].
Now,
Again for the region \[{x_2}\] to \[{x_3}\], $F$ is positive.
Thus, we can say $dU$ is negative.
Which means that potential energy decreases from \[{x_2}\] to \[{x_3}\].
Now,
For the region \[{x_3}\] to \[{x_4}\] , the force keeps on decreasing making it negative.
Thus,
$F$ is negative here.
Thus,
\[dU\] is positive.
Which means potential energy increases here.
Thus, taking all these observations into consideration, we can say (A) is the correct option.
Note: When $dU$ is positive, the potential energy increases because $dU$ refers to the change in the potential energy. When its value is positive, the final potential energy value is greater than the initial potential energy value. Thus making its overall value to be positive. The same analogy goes true when $dU$ is negative.
Formulae Used $F = - \frac{{dU}}{{dx}}$
Step By Step Solution
Firstly,
$F = - \frac{{dU}}{{dx}}$
Thus, we can say,
\[dU = - Fdx\]
Now,
Clearly,
When $F$ is positive, $dU$ is negative and when $F$ is negative, $dU$ is positive.
For the region from \[{x_1}\] to \[{x_2}\] , $F$ is negative as we can see from the graph itself.
Thus, $dU$ is positive.
Which means potential energy increases from \[{x_1}\] to \[{x_2}\].
Now,
Again for the region \[{x_2}\] to \[{x_3}\], $F$ is positive.
Thus, we can say $dU$ is negative.
Which means that potential energy decreases from \[{x_2}\] to \[{x_3}\].
Now,
For the region \[{x_3}\] to \[{x_4}\] , the force keeps on decreasing making it negative.
Thus,
$F$ is negative here.
Thus,
\[dU\] is positive.
Which means potential energy increases here.
Thus, taking all these observations into consideration, we can say (A) is the correct option.
Note: When $dU$ is positive, the potential energy increases because $dU$ refers to the change in the potential energy. When its value is positive, the final potential energy value is greater than the initial potential energy value. Thus making its overall value to be positive. The same analogy goes true when $dU$ is negative.
Recently Updated Pages
Average fee range for JEE coaching in India- Complete Details

Difference Between Rows and Columns: JEE Main 2024

Difference Between Length and Height: JEE Main 2024

Difference Between Natural and Whole Numbers: JEE Main 2024

Algebraic Formula

Difference Between Constants and Variables: JEE Main 2024

Trending doubts
Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Physics Average Value and RMS Value JEE Main 2025

Charging and Discharging of Capacitor

Electromagnetic Waves Chapter - Physics JEE Main

Collision - Important Concepts and Tips for JEE

A pressure of 100 kPa causes a decrease in volume of class 11 physics JEE_Main

Other Pages
NCERT Solutions for Class 11 Physics Chapter 4 Laws of Motion

NCERT Solutions for Class 11 Physics Chapter 7 Gravitation

NCERT Solutions for Class 11 Physics In Hindi Chapter 1 Physical World

NCERT Solutions for Class 11 Physics Chapter 14 Waves

JEE Advanced 2025 Notes

A water pump of 1 horse power is required to flow water class 11 physics JEE_Main




